Happiness
Chess, Happiness, and Chance
If you can’t win most games, can you still be happy?
Posted April 14, 2024 Reviewed by Gary Drevitch
Key points
- Playing chess arouses ambivalent emotions.
- One may not expect to win most games.
- Some psychological tricks may increase happiness, at least in the short term.
This post was written with Nada Saaidia and David J. Grüning.
Chess is mental torture. — Garry Kasparov
Why play chess if it is torture, as Kasparov testified? One view is that chess is a social trap leading its victims into obsession. It’s hard work but it's fun to learn the ropes and to begin scoring victories with elegant moves, stealthy gambits, and Zugzwang (compelling the opponent to make one move that leads to their checkmate). In time, the ardent learner will tire of smashing novices; she will look for opponents in her own league. With more time, she will realize that she loses about half of her games. On average, it has to be so. Setting aside games ending in a draw, half are won and half are lost. It has to be so. More importantly, this will be so for most individual players. At the fringes of the distribution, we may find masters enjoying themselves beating up the weaklings, and novices seeking the lessons of defeat by challenging masters. But generally, when talent and skill are grossly mismatched, no one is happy. Hence, most players face opponents at their own skill level, and must make peace with the fact that they will lose as often as they win.
Whence happiness? The addictive potential of chess may be amplified in the online playing world, on platforms like chess.com. Some readers may identify with the feeling of still being up at 2 a.m., bleary-eyed and exhausted, promising themselves that it will be just one more game. We will set this descent into sadness aside and focus on days with a reasonable number of games played, to allow us to maintain the assumption that the probability of winning is .5 for each game. Hot hands may exist but again, let’s simplify.
How might a player extract some happiness from daily play? It occurred to one of us (JIK) that it might be a fine idea to play each day until a game was won. The expectation was that on half of the days, only one game would be played. On a quarter of play days, two games would be played; on an eighth of days, three games would be played, and so forth. A victory would deliver one positive hedon, whereas a defeat would deliver one negative hedon. We further simplify by assuming that the pleasure of victory is as intense as the pain of defeat.
Is this self-regulatory rule conducive to happiness? JIK’s intuition was that it would be. He noted that every other day would be a happy one, marked by the one won game. Days with multiple defeats become geometrically less likely, so why bother? Rather than overestimating small probabilities, one might neglect them altogether, or so he thought (Krueger & Grüning, 2024). The other hint toward happiness was the fact that every day, no matter the length of the (improbable) string of defeats, would end with a victory, and Kahneman’s peak-end-rule of pain memory, paired with duration neglect, says that the last event dominates mood and memory, whereas the drought of defeats preceding it is not made worse by increasing length (Kahneman, 2000). In short, JIK’s intuition was anchored in biased probability beliefs and biased memory for events in a sequence.
DJG had the opposing intuition. He made it clear that he would rather play until a game was lost. This means every other day would bring only one painful event, but there could be some (though improbable) glorious days with multiple victories. This intuition also finds friends in the psychological literature—to wit, it is consistent with range-frequency thinking (Parducci, 1965) which suggests that the frequency of positive events drives overall happiness.
Both intuitions are conditioned on an arbitrary mental shortcut: the segmentation of the overall sequence of games by day. To be sure, a player must sleep, and this gives some sense to this segmentation. Aftershocks of the day’s experiences at the board may resonate during the brain’s alpha-wave cycle. The logician and the statistician, however, will object. Let us be clear, they will note that the total sequence of games is what a player can pull off over a lifetime, and that means that when the end nears, they can look back on 50% of games won and 50% lost (again, setting aside draws and the exquisitely frustrating stalemates—frustrating for the near-winner, that is, as explainable by norm theory; Kahneman & Miller, 1986).
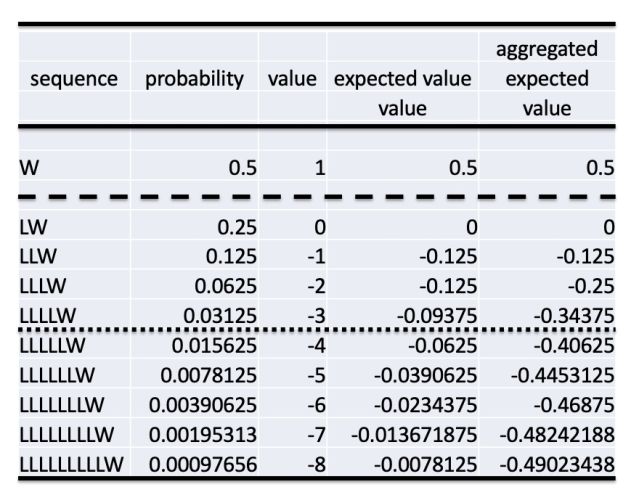
The original impetus for this essay was JIK’s (false) intuition that the daily playing rule of stopping after a victory could cheat that which is probabilistically inevitable in the long run. To remind ourselves how this intuition came about, let us look at the matrix above in which W refers to a win and L to a loss. The longer a sequence becomes—due to more losses endured before a win is enjoyed—the less probable it is. The expected value of a sequence is the product of the sum of hedons (+1 for a W and -1 for an L) and its probability. The aggregated expected value for all days beginning with a loss is the sum of the expected values for all sequences up to the longest one being considered. As we can see, when four losing sequences are considered, the aggregated expected value (-.34) is .16 points lower in absolute terms than the expected value of days ending in one win (i.e., +.5). JIK’s error was to think that it would just take too long for losses to undo the benefit of the wins, if it ever happened at all.
A moment of reflection, following the realization that the daily segmentation is arbitrary, forces the recognition that the law of large numbers guarantees equally extreme expected values, and thus the cancellation of wins by losses (Stigler, 1986). In the long run, the total expected value is 0. It should be enough to remember that the probability of a win is p = .5, that the probability of a loss is 1 – p, and that a win brings +1 hedon of happiness and that a loss brings -1 hedon. As we move down in the matrix past the dotted line, we see that the aggregated expected value of nine loss-bearing sequences almost reaches the expected value of a one-win day in absolute terms. One of us, NS, produced a beautiful mathematical proof. A bit of algebra and differentiation shows what has to be so. The proof is available to those who request it.
Meanwhile, JIK has looked for other ways to escape the inevitable. With a modicum of pangs of conscience, he thought he might throw a bunch of games to lower his skill score, to then be paired with opponents who won’t resort to such shenanigans, and to enjoy victories with p > .5. Yet, happiness remains elusive.
References
Kahneman, D. (2000). Evaluation by moments: Past and future. In D. Kahneman & A. Tversky (eds.), Choices, values, and frames (pp. 693-708). New York, NY: Cambridge University Press.
Kahneman, D., & Miller, D. T. (1986). Norm theory: Comparing reality to its alternatives. Psychological Review, 93, 136–153.
Krueger, J. I., & Grüning, D. J. (2024). Dostoevsky at play: Between risk and uncertainty in Roulettenburg. In S. Evdokimova (ed). Dostoevsky’s The Gambler: The allure of the wheel (pp. 59-86). Lexington Books.
Parducci, A. (1965). Category judgment: A range-frequency model. Psychological Review, 72\, 407–418.
Stigler, S. M. (1986). The history of statistics: The measurement of uncertainty before 1900. Cambridge, MA: The Belknap Press of the Harvard University Press.


